What is a cube net?
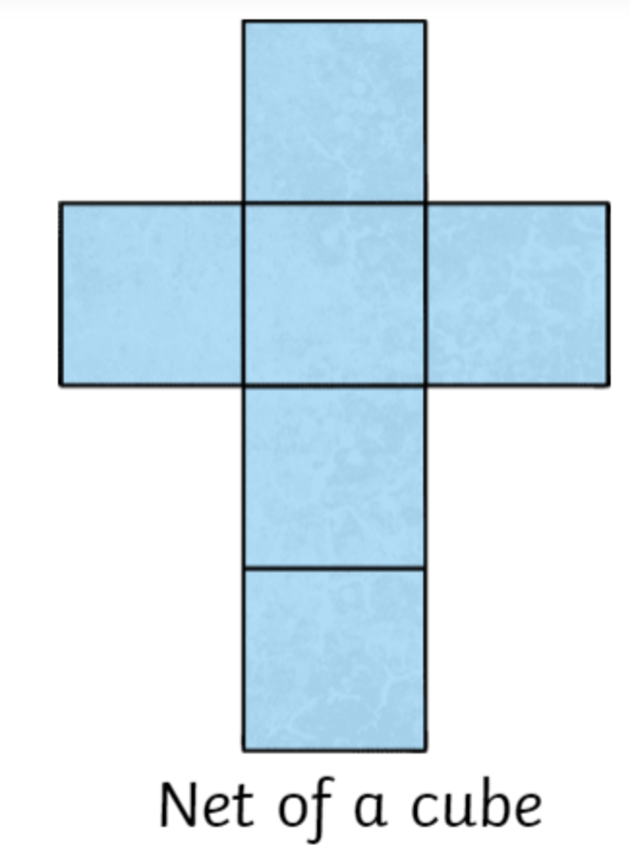
A cube net is a two-dimensional representation of a three-dimensional cube. It is made up of six squares, each of which represents one side of the cube. The squares are connected by lines, which represent the edges of the cube.
Cube nets are used for a variety of purposes, including:
- Creating three-dimensional models of cubes
- Calculating the surface area and volume of cubes
- Understanding the geometry of cubes
Cube nets are an important tool for understanding three-dimensional shapes. They can be used by students, teachers, and anyone else who wants to learn more about geometry.
Cubes Net
Cubes nets are two-dimensional representations of three-dimensional cubes. They are essential for understanding the geometry of cubes and for creating three-dimensional models.
- Definition: A cube net is a two-dimensional representation of a three-dimensional cube.
- Construction: Cube nets are made up of six squares, each of which represents one side of the cube.
- Edges: The squares are connected by lines, which represent the edges of the cube.
- Vertices: The points where the lines intersect are called vertices.
- Faces: The squares that make up the cube net are called faces.
- Dimensions: The dimensions of a cube net are the length, width, and height of the cube.
1. Definition
This definition establishes the fundamental relationship between a cube net and the three-dimensional cube it represents. A cube net is like a blueprint or template that can be used to construct a three-dimensional cube.
- Red Funyuns A Tasty Treat For All Ages
- Discover The Rarest Gold Penny Of Them All A Treasure From History
- Components: A cube net is made up of six squares, each of which represents one face of the cube. The squares are connected by lines, which represent the edges of the cube.
- Construction: To construct a three-dimensional cube from a cube net, the squares are folded along the lines and taped or glued together. This process is similar to folding a paper box from a two-dimensional template.
- Example: A common example of a cube net is the unfolded box of a Rubik's Cube. The six faces of the cube are printed on the squares of the net, and the lines represent the creases where the box is folded.
Cube nets are an important tool for understanding the geometry of cubes. They can be used to calculate the surface area and volume of cubes, and to create three-dimensional models of cubes. Cube nets are also used in a variety of applications, such as packaging and architecture.
2. Construction
This statement defines the construction of a cube net, highlighting that it consists of six squares, each representing a side of the cube. This construction is crucial for understanding the properties and applications of cube nets.
The six squares that make up a cube net are connected by lines, which represent the edges of the cube. This structure allows the cube net to be folded into a three-dimensional cube, with each square becoming a face of the cube. The edges of the squares become the edges of the cube, and the vertices where the lines intersect become the vertices of the cube.
Understanding the construction of cube nets is essential for a variety of applications. For example, cube nets are used in packaging to create boxes and containers. They are also used in architecture to design buildings and structures. By understanding how cube nets are constructed, engineers and architects can create efficient and stable designs.
In summary, the construction of cube nets, consisting of six squares representing the sides of the cube, is fundamental for understanding the geometry of cubes and their practical applications.
3. Edges
In the context of cube nets, edges play a crucial role in defining the shape and structure of the three-dimensional cube.
- Defining the Cube's Shape: The edges of a cube net determine the shape of the resulting cube. By connecting the squares along the edges, the net forms a three-dimensional structure, giving the cube its characteristic square faces and right angles.
- Ensuring Structural Stability: The edges of a cube net act as the framework that holds the cube together. When the net is folded and assembled, the edges provide support and prevent the cube from collapsing.
- Calculating Cube Dimensions: The lengths of the edges are directly related to the dimensions of the cube. By measuring the length of an edge, one can determine the length, width, and height of the corresponding cube.
- Understanding Cube Geometry: The edges of a cube net help visualize and understand the geometry of cubes. By examining the angles and relationships between the edges, one can gain insights into the properties of cubes and other three-dimensional shapes.
In summary, the edges of a cube net play a vital role in shaping, stabilizing, measuring, and understanding the geometry of cubes. They are essential components that contribute to the functionality and significance of cube nets.
4. Vertices
In the context of cube nets, vertices play a significant role in defining the cube's structure and properties.
Vertices are the points where the edges of a cube net intersect. Each vertex represents a corner of the resulting cube. The number of vertices in a cube net is equal to the number of corners in a cube, which is eight.
Vertices are crucial for understanding the geometry of cubes. By examining the angles and relationships between the vertices, one can gain insights into the cube's shape, dimensions, and spatial orientation.
For example, in architecture, understanding the vertices of a cube net is essential for designing and constructing buildings and structures. Architects use cube nets to visualize and plan the layout of rooms, windows, and other architectural elements.
In summary, vertices area fundamental component of cube nets, providing insights into the geometry and properties of cubes. Understanding the role of vertices is essential for a variety of applications, including architecture, engineering, and mathematics.
5. Faces
Faces are an integral part of cube nets, forming the basic structure and defining the properties of the resulting cube.
The faces of a cube net are the squares that make up its surface area. When the net is folded into a three-dimensional cube, the faces become the sides of the cube. The number of faces in a cube net is equal to the number of sides in a cube, which is six.
Understanding the faces of a cube net is crucial for visualizing and comprehending the geometry of cubes. By examining the shape, size, and arrangement of the faces, one can gain insights into the cube's dimensions, angles, and spatial relationships.
In practical applications, the faces of cube nets play a significant role. For example, in packaging design, the faces of a cube net determine the size, shape, and appearance of the resulting box or container. In architecture, the faces of a cube net help architects plan the layout and design of buildings and structures.
In summary, the faces of a cube net are essential components that contribute to the structural integrity, geometric properties, and practical applications of cube nets.
6. Dimensions
The dimensions of a cube net are closely related to the dimensions of the cube it represents. The length, width, and height of the cube net are directly proportional to the length, width, and height of the cube, respectively.
Understanding the dimensions of a cube net is crucial for several reasons. First, it allows us to determine the size and scale of the cube that will be constructed from the net. Second, it helps us to calculate the surface area and volume of the cube. Third, it enables us to compare different cube nets and select the one that is most suitable for a particular application.
In practical applications, the dimensions of cube nets play a significant role. For example, in packaging design, the dimensions of a cube net determine the size and shape of the resulting box or container. In architecture, the dimensions of a cube net help architects plan the layout and design of buildings and structures.
In summary, understanding the dimensions of cube nets is essential for a variety of applications. It allows us to determine the size, scale, surface area, and volume of the cube that will be constructed from the net. This understanding is crucial for designing and constructing objects and structures in the real world.
FAQs about Cube Nets
Cube nets are two-dimensional representations of three-dimensional cubes. They are used for a variety of purposes, including creating three-dimensional models of cubes, calculating the surface area and volume of cubes, and understanding the geometry of cubes.
7. 1. What is a cube net?
A cube net is a two-dimensional representation of a three-dimensional cube. It is made up of six squares, each of which represents one side of the cube. The squares are connected by lines, which represent the edges of the cube.
8. 2. How do you make a cube net?
To make a cube net, start by drawing a square. Then, draw four more squares around the first square, each sharing a side with the first square. Finally, draw lines connecting the corresponding vertices of the squares.
9. 3. What is the surface area of a cube net?
The surface area of a cube net is the sum of the areas of the six squares that make up the net. The area of a square is equal to the length of one side squared. Therefore, the surface area of a cube net is equal to 6 * (length of one side)2.
10. 4. What is the volume of a cube net?
The volume of a cube net is equal to the length of one side cubed. This is because the volume of a cube is equal to the length of one side cubed, and a cube net can be folded into a cube.
11. 5. What are cube nets used for?
Cube nets are used for a variety of purposes, including:
- Creating three-dimensional models of cubes
- Calculating the surface area and volume of cubes
- Understanding the geometry of cubes
- Packaging
- Architecture
Conclusion
Cube nets are a fundamental tool for understanding the geometry of cubes. They can be used to create three-dimensional models of cubes, calculate the surface area and volume of cubes, and understand the relationships between the different parts of a cube.
Cube nets are also used in a variety of practical applications, such as packaging and architecture. By understanding the properties of cube nets, engineers and architects can design efficient and stable structures.
In conclusion, cube nets are a versatile and important tool for a variety of applications. Their ability to represent three-dimensional objects in two dimensions makes them a powerful tool for understanding and manipulating the world around us.
Detail Author:
- Name : Mrs. Jane Wyman I
- Username : clare18
- Email : natalie38@baumbach.org
- Birthdate : 1985-11-09
- Address : 486 Mohammad Causeway Kreigershire, DC 17788
- Phone : 585.959.0381
- Company : Conn, Bartell and Bergnaum
- Job : Ship Pilot
- Bio : Pariatur harum deserunt voluptatibus ipsa reprehenderit omnis asperiores. Eaque atque voluptatibus sit sequi. Dignissimos iusto eos excepturi dolorem et voluptas illo quis.
Socials
instagram:
- url : https://instagram.com/hassie_dev
- username : hassie_dev
- bio : Et ut voluptatum fugit officiis. Soluta corrupti aperiam sed eaque. Esse non est fuga dolorem et.
- followers : 1803
- following : 2031
facebook:
- url : https://facebook.com/hdach
- username : hdach
- bio : Eveniet vel quia sunt magni enim totam fugit.
- followers : 5009
- following : 640
linkedin:
- url : https://linkedin.com/in/hassie.dach
- username : hassie.dach
- bio : Aperiam recusandae ut et iste.
- followers : 3302
- following : 2572
twitter:
- url : https://twitter.com/hassiedach
- username : hassiedach
- bio : Sit nihil enim omnis esse doloremque. Nihil neque voluptatem ullam. Qui enim in vel et.
- followers : 4036
- following : 2168
tiktok:
- url : https://tiktok.com/@dachh
- username : dachh
- bio : Qui aut voluptas voluptatem maiores harum iure.
- followers : 4876
- following : 1574